Understanding "Bending Modes"
- mcfateconsulting
- Apr 10, 2017
- 3 min read
I'm hoping to initiate a series of articles dealing with various topics in the Machine Condition Monitoring space. The intent is to keep most of these pretty short, and as entertaining as possible.
I have laid a little groundwork and now I’ll tie together a couple of concepts. The last article on Resonance established that everything will have a particular resonant frequency. It’s a little more complicated than this, because a component will not have only ONE frequency. There are actually multiple frequencies at which a single component of any machine will vibrate.
The base cause of this is that any component will have several BENDING MODES. This is most easily visualized with the ‘string’ analogy as most people at least know what a guitar of a violin looks like, and it’s also is sorta two-dimensional and easier to wrap your head around. As described previously, in our string example the two factors that determine resonant frequency are the MASS, and the STIFFNESS of the string. Casual inspection of a guitar or other stringed instrument first reveals that the strings are NOT all the same diameter. The low notes strings are noticeably thicker than the high note strings. This affects the MASS of the system. Greater mass results in a lower resonant frequency, and lower mass makes the resonant frequency higher. The string is rigidly secured at both ends, but one end has a mechanical ‘tuning key’ that can be used to tighten or loosen tension on the string. This allows the STIFFNESS to be adjusted to ‘tune’ the instrument. Now, let’s just consider only one of these multiple strings on the guitar. We adjust the tension to get the ‘open’ frequency desired. From this point onward, the basic equation, mass and stiffness, is satisfied, and we get a pleasing “E” note. The movement of the string as it vibrates is a full-length oscillation with the maximum deflection in the exact center of the length… this is the FIRST BENDING MODE of the string. It is essentially one-half of a complete sine wave.

By lightly placing a finger at the exact center of the string we prevent the middle of the string from moving, Plucking the string again causes the string to vibrate between the three ‘fixed’ points in the system, and the string now vibrates as a full sine wave, the frequency of resonance DOUBLES. The octave (another term we’ll discuss later) fret on the instrument is the exact center of the length of the string, and corresponds to the SECOND BENDING MODE, and a full doubling of the vibration frequency.

Moving our finger to a position 1/3 the length of the string and plucking causes the string to vibrate in a shape that is 1 and ½ sine – THIRD BENDING MODE.
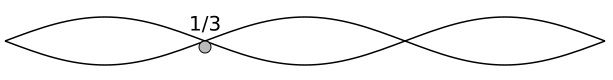
This progression continues for higher modes, FOURTH, FIFTH, ect. Without changing the mass or the stiffness of the ‘system'. Understanding this, it is simple to imagine the various frequencies that exist in a structure, and there are a BUNCH of them! Any of these basic modes can be forced into resonance by a driving force at the same frequency. Generally, higher modes are more difficult to excite, and will require more energy to force them into resonance. However, you can get things moving with the right (or perhaps the WRONG) driving forces.






















Comments